Trees

• a special type of graph
• trees excel at storing data hierarchically and are commonly used as a means of testing your knowledge of recursion during coding interviews
• it is a data structure that consists of nodes, each with some value and pointers to child-nodes
  • which recursively form subtrees in the tree
• the 1st node in a tree is referred to as the root of the tree
  • while the nodes at the bottom of a tree (the nodes with no child-nodes) are referred to as leaf nodes or leaves
  • the paths between the root of a tree and its leaves are called branches
  • the height of a tree is the length of its longest branch
  • the depth of a tree node is its distance from its tree's root
    • also known as the node's level in the tree
• a tree is effectively a graph that's connected, directed, and acyclic
  • has an explicit root node, and whose nodes all have a single parent (except for the root node)
  • in most implementations of trees, tree nodes don't have a pointer to their parent, but can if desired

Types of trees

Binary Trees

• the root node has 2 child nodes
• every other nodes have up to 2 child nodes
• the structure of a binary tree is such that many of its operations have a logarithmic time complexity, making binary tree a commonly used data structure

class Node:
  def __init__(self, value):
    self.value = value
    self.left = None
    self.right = None

class Node<T> {
  constructor(public value: T) {
    this.value = value;
    this.left = null;
    this.right = null;
  }
}

Binary Search Tree (BST)

• it is a node-based binary tree data structure which has the following properties

  • The left subtree of a node contains only nodes with values smaller than the node's value
  • The right subtree of a node contains only nodes with values bigger than the node's value
  • The left and right subtree each must also be a binary search tree or be null

• If a node is to be inserted into a tree, it is compared with current node (starting from the root)

  • If the node's value is less than current, we send it down to left subtree
  • If node's value is greater than or equal to current
    • it is sent to the right subtree
    • This process is repeated for each encountered node

K-ary Tree

• a tree where every node (including the root) have up to k child nodes
  • e.g.: a binary tree is a k-ary tree where k === 2

Perfect Binary Tree

• a binary tree whose interior nodes all have 2 child nodes and whose leaf nodes all have the same depth

          1
      /         \
     2           3
    /  \       /   \
   4    5     6     7
   /\   /\    /\    /\
  8  9 10 11 12 13 14 15

Complete Binary Tree

• a binary tree that's almost perfect
• its interior nodes all have 2 child nodes
• but its leaf nodes don't necessarily all have the same depth
• furthermore, the nodes in the last level of a complete binary tree are as far left as possible

        1
      /   \
     2     3
    / \   / \
   4   5 6   7
   /\
  8  9
• a binary tree is incomplete if the nodes in its last level aren't as far left as possible

        1
      /   \
     2     3
    / \   / \
   4   5 6   7
      /   \
     8     9

Balanced Binary Tree

       1
     /    \
    2      3
   / \    / \
  4   5  6   7
 / \        /
10  9      8

• a binary tree whose nodes all have left and right subtrees whose heights differ by no more than 1
• a balanced binary tree is such that the logarithmic time complexity O(log n) of its operations is maintained
• e.g.: inserting a node at the bottom of the following imbalanced binary tree's left subtree would clearly not be a logarithmic-time operation, since it would involve traversing through most of the tree's nodes

           1
         /  \
        2    3
       /
      4
     /
    8
   /
  10

Full Binary Tree

• a binary tree whose nodes all have either 2 child nodes or 0 child nodes

    1
   / \
  2   3
     / \
    6   7
   / \
  8   9

Heaps

• they are typically binary heaps
• a special type of binary trees, where every node in the tree satisfies the min or max heap property

Min Heaps

Max Heaps

Ternary Tree

• the root node has 3 child nodes
• every other nodes have up to 3 child nodes

Tries

• a tree like data structure that typically stores characters in a string

AVL Trees

• able to rebalance themselves to obtain the log N complexity

Red Black Trees

• able to rebalance themselves to obtain the log N complexity

standard operations and complexities

Storing all types of tree: O(N) space

• N is the total number of nodes in the tree

Traversing through the entire tree: O(N) time

Traversing 1 subtree at every step for a balance binary tree: O(log N) time on average

• if the tree is skewed, it becomes O(N) time on worst

Search, Insert, Delete

• for Binary Search Tree: O(h)
  • h is the height of the BST
• for skewed tree: O(n)
  • n is the number of nodes

Traversal Types

• Using Recursion

pros	cons
The code may be easier to write	Recursive functions are generally slower than non-recursive function
To solve such problems which are naturally recursive such as tower of Hanoi	It may require a lot of memory space to hold intermediate results on the system stacks
Reduce unnecessary calling of function	Hard to analyze or understand the code
Extremely useful when applying the same solution	It is not more efficient in terms of space and time complexity
Recursion reduce the length of code	The computer may run out of memory if the recursive calls are not properly checked
It is very useful in solving the data structure problem
Stacks evolutions and infix, prefix, postfix evaluations etc

Breath First Traversal

Depth First Traversal

Inorder (Left, Root, Right)

• example
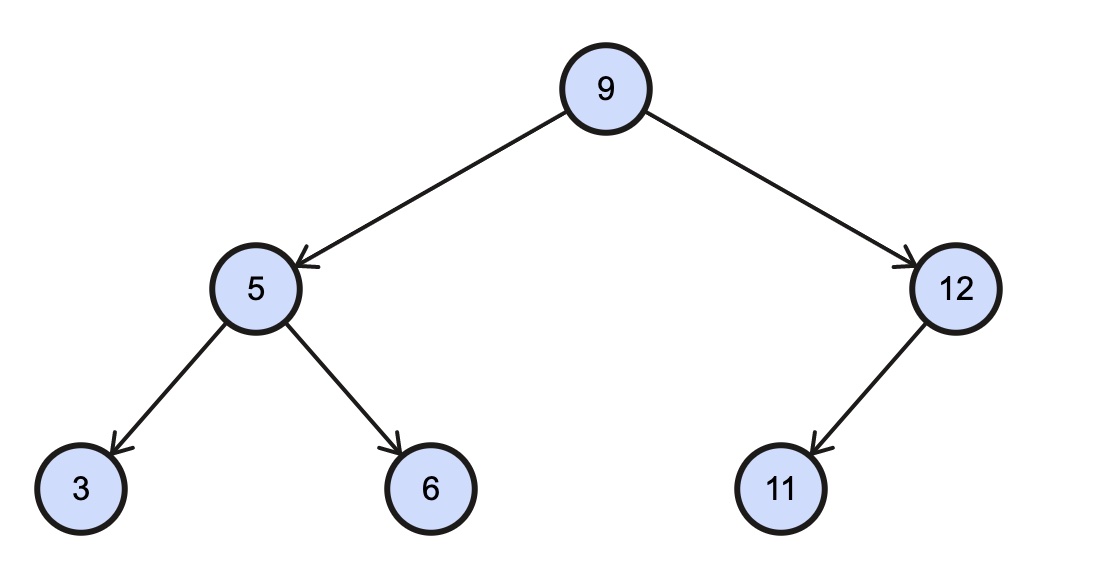
  • 3, 5, 6, 9, 11, 12
• prints the values in the left subtree, then the value of the root subtree, then the right subtree

1. Traverse the left subtree, i.e., call Inorder(left-subtree)
2. Visit the root.
3. Traverse the right subtree, i.e., call Inorder(right-subtree)

def in_order(current_node):
  if current_node:
    in_order(current_node.left)
    print(current_node.value)
    in_order(current_node.right)

function inOrder(node: Node | null) {
  if (node) {
    inOrder(node.left);
    console.log(node.value);
    inOrder(node.right);
  }
}

def in_order(root):
  if root is None:
    return

  node_stack = []
  node_stack.append(root)

  while(len(node_stack) > 0):
    current_node = node_stack.pop()

    # Note that right child is pushed first so that left is processed first
    if current_node.right:
      node_stack.append(current_node.right)

    print(current_node.value)

    if current_node.left:
      node_stack.append(current_node.left)

Preorder (Root, Left, Right)

• example
  • 9, 5, 3, 6, 12, 11
• prints the root value, then value in left subtree, then value in right subtree

1. Visit the root.
2. Traverse the left subtree, i.e., call Preorder(left-subtree)
3. Traverse the right subtree, i.e., call Preorder(right-subtree)

def pre_order(current_node):
  if current_node:
    print(current_node.value)
    pre_order(current_node.left)
    pre_order(current_node.right)

function preOrder(node: Node | null) {
  if (node) {
    console.log(node.value);
    preOrder(node.left);
    preOrder(node.right);
  }
}

def pre_order(root):
  if root is None:
    return

  node_stack = []
  node_stack.append(root)

  while(len(node_stack) > 0):
    current = node_stack.pop()
    print(current.value)

    # Note that right child is pushed first so that left is processed first
    if current.right:
      node_stack.append(current.right)
    if current.left:
      node_stack.append(current.left)

Postorder (Left, Right, Root)

• example
  • 3, 6, 5, 11, 12, 9
• prints the value of the left subtree, then the value of the right subtree, then the root value

1. Traverse the left subtree, i.e., call Postorder(left-subtree)
2. Traverse the right subtree, i.e., call Postorder(right-subtree)
3. Visit the root.

def post_order(current_node):
  if current_node:
    post_order(current_node.left)
    post_order(current_node.right)
    print(current_node.value)

function postOrder(node: TreeNode | null) {
  if (node) {
    postOrder(node.left);
    postOrder(node.right);
    console.log(node.value);
  }
}

def post_order(root):
  if root is None:
    return

  node_stack = []
  node_stack.append(root)

  while(len(node_stack) > 0):
    current = node_stack.pop()

    # Note that right child is pushed first so that left is processed first
    if current.right:
      node_stack.append(current.right)
    if current.left:
      node_stack.append(current.left)

    print(current.value)

Binary Search

def binary_search(current, target):
  if current is None:
    return None

  if current.value == target:
    return current

  if target > current.value:
    return binary_search(current.right, target)

  if target < current.value:
    return binary_search(current.left, target)

  return None

function binarySearch(current: Node | null, target: number): Node | null {
  if (current === null) {
    return null;
  }

  if (target === current.value) {
    return current;
  }

  if (target > current.value) {
    return binarySearch(current.right, target);
  }

  if (target < current.value) {
    return binarySearch(current.left, target);
  }

  return null;
}

def binary_search(tree, target):
  current = tree
  while current:
    if current.value == target:
      return current.value
    if target > current.value:
      current = current.right
    elif target < current.value:
      current = current.left
    else:
      break
  return None

Min and Max of Binary Search Trees

Min

• value is a minimum by following left child pointers from the root until we encounter a null

function treeMinimum(node: Node) {
  while (node.left) {
    node = node.left;
  }

  return node;
}

Max

• value is a maximum by following right child pointers from the root until we encounter a null

function treeMaximum(node: Node) {
  while (node.right) {
    node = node.right;
  }

  return node;
}

Successor

• it is the node with the smallest value greater than targetNode.value

function getSuccessor(tree: Node, targetNode: Node) {
  if (tree === targetNode) {
    return null;
  }

  if (targetNode.right) {
    return treeMinimum(targetNode.right);
  }

  let successor = null;
  let currentNode: Node | null = tree;

  while (currentNode) {
    if (currentNode.value <= targetNode.value) {
      currentNode = currentNode.right;
    } else {
      successor = currentNode;
      currentNode = currentNode.left;
    }
  }

  return successor;
}

Insertions

function insertNode(tree: Node | null, node: Node) {
  if (!tree) {
    return node;
  }

  let leafNode: Node | null = tree;
  let leafNodeParent: Node = tree;

  while (leafNode) {
    leafNodeParent = leafNode;

    leafNode = node.value < leafNode.value ? leafNode.left : leafNode.right;
  }

  if (node.value < leafNodeParent.value) {
    leafNodeParent.left = node;
  } else {
    leafNodeParent.right = node;
  }
}

Deletion

• The overall strategy for deleting a node node from a binary search tree tree has three basic cases

  • If node is leaf (has no children)

    • then remove it by modifying its parent to replace node with null as its child

    

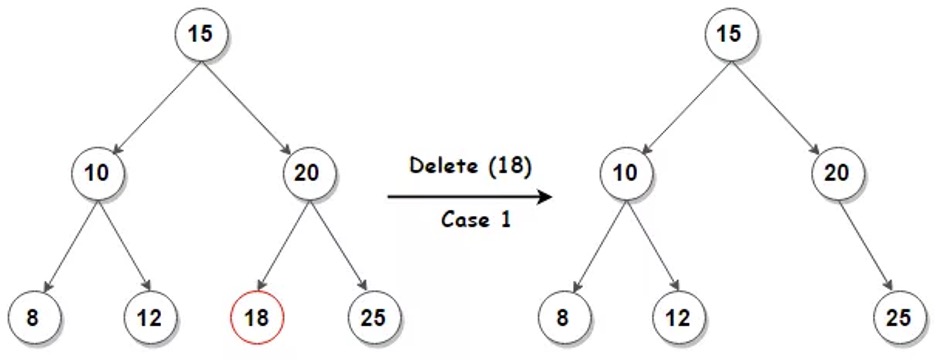
  • If node has just one child

    • then elevate that child to take nodes position in the tree by modifying node parent to replace node by nodes child

    

  • If node has two children

    • then find node successor parent
      • must be in node right subtree
      • and have parent take nodes position in the tree
    • The rest of nodes original right subtree becomes parents new right subtree, and nodes left subtree becomes parents new left subtree
    • This case is the tricky because it matters whether parent is nodes right child

    

function deleteNode(tree: Node | null, value: number): Node | null {
  if (tree === null) {
    return tree;
  }

  if (value < tree.value) {
    tree.left = deleteNode(tree.left, value);
  } else if (value > tree.value) {
    tree.right = deleteNode(tree.right, value);
  }
  // this is the node to be deleted
  else {
    // node with only one child or no child
    if (tree.left === null) {
      return tree.right;
    }

    if (tree.right === null) {
      return tree.left;
    }

    // node with two children: get the inorder predecessor (largest in the left subtree)
    tree.value = treeMaximum(tree.left).value;

    // delete the inorder predecessor
    tree.left = deleteNode(tree.left, tree.value);
  }

  return tree;
}

• full Binary Search Tree example

  • utils

    enum Compare {
      LessThan = -1,
      BiggerThan = 1,
      Equals = 0,
    }
    
    function compare<T>(a: T, b: T): number {
      if (a === b) {
        return Compare.Equals;
      }
      return a < b ? Compare.LessThan : Compare.BiggerThan;
    }

  export class Node<T> {
    public left: Node<T> = null;
    public right: Node<T> = null;
  
    constructor(public value: T) {
      this.value = value;
    }
  
    public toString() {
      return `${this.value}`;
    }
  }

  class BinarySearchTree<T> {
    protected root: Node<T> = null;
  
    /**
     * @description: insert element
     */
    public insert(value: T) {
      if (this.root === null) {
        // edge case: insert into root node
        this.root = new Node(value);
      } else {
        // recursively find the insertion position
        this.insertNode(this.root, value);
      }
    }
  
    /**
     * @description: recursive insertion method
     */
    protected insertNode(node: Node<T>, value: T) {
      if (compare(value, node.value) === Compare.LessThan) {
        // If the value is smaller than node.value, go to the left
        if (node.left === null) {
          // Baseline condition: the left side is empty and directly assigned
          node.left = new Node(value);
        } else {
          // otherwise recurse
          this.insertNode(node.left, value);
        }
        // If the value is larger than node.value, go to the right
      } else if (node.right === null) {
        // Baseline condition: the right side is empty and directly assigned
        node.right = new Node(value);
      } else {
        // otherwise recurse
        this.insertNode(node.right, value);
      }
    }
  
    /**
     * @description: return the root node
     */
    public getRoot() {
      return this.root;
    }
  
    /**
     * @description: search element
     */
    public search(value: T) {
      // call recursive search element method
      return this.searchNode(this.root, value);
    }
  
    /**
     * @description: recursive search element method
     */
    private searchNode(node: Node<T>, value: T): boolean {
      // Baseline condition: return false when null is found
      if (node === null) {
        return false;
      }
  
      if (compare(value, node.value) === Compare.LessThan) {
        // value is smaller than node.value, look left
        return this.searchNode(node.left, value);
      } else if (compare(value, node.value) === Compare.BiggerThan) {
        // value is bigger than node.value, look right
        return this.searchNode(node.right, value);
      }
      // value is equal to node.value, element is found
      return true;
    }
  
    public inOrderTraverse(callback: Function) {
      this.inOrderTraverseNode(this.root, callback);
    }
  
    private inOrderTraverseNode(node: Node<T>, callback: Function) {
      if (node !== null) {
        this.inOrderTraverseNode(node.left, callback);
        callback(node.value);
        this.inOrderTraverseNode(node.right, callback);
      }
    }
  
    public preOrderTraverse(callback: Function) {
      this.preOrderTraverseNode(this.root, callback);
    }
  
    private preOrderTraverseNode(node: Node<T>, callback: Function) {
      if (node !== null) {
        callback(node.value);
        this.preOrderTraverseNode(node.left, callback);
        this.preOrderTraverseNode(node.right, callback);
      }
    }
  
    postOrderTraverse(callback: Function) {
      this.postOrderTraverseNode(this.root, callback);
    }
  
    private postOrderTraverseNode(node: Node<T>, callback: Function) {
      if (node !== null) {
        this.postOrderTraverseNode(node.left, callback);
        this.postOrderTraverseNode(node.right, callback);
        callback(node.value);
      }
    }
  
    /**
     * @description: return smallest element in tree
     */
    public min() {
      // call iterator method
      return this.minNode(this.root);
    }
  
    /**
     * @description: returns the smallest element under the specified subtree
     */
    protected minNode(node: Node<T>) {
      let current = node;
  
      // keep looking left
      while (current !== null && current.left !== null) {
        current = current.left;
      }
      return current;
    }
  
    /**
     * @description: return largest element in tree
     */
    public max() {
      // call iterator method
      return this.maxNode(this.root);
    }
  
    /**
     * @description: returns the largest element under the specified subtree
     */
    protected maxNode(node: Node<T>) {
      let current = node;
  
      // keep looking right
      while (current !== null && current.right !== null) {
        current = current.right;
      }
      return current;
    }
  
    /**
     * @description: remove the specified element
     */
    public remove(value: T) {
      // Call the recursive method, the recursion here is special, and the deleted tree will be returned
      this.root = this.removeNode(this.root, value);
    }
  
    /**
     * @description: A recursive method that removes the specified element in the specified subtree. After each processing, the processed node needs to be returned to this node
     */
    protected removeNode(node: Node<T>, value: T) {
      // baseline condition
      if (node === null) {
        return null;
      }
  
      if (compare(value, node.value) === Compare.LessThan) {
        // When the value is less than node.value, look left
        node.left = this.removeNode(node.left, value);
        return node;
      } else if (compare(value, node.value) === Compare.BiggerThan) {
        // When the value is greater than node.value, look to the right
        node.right = this.removeNode(node.right, value);
        return node;
      } else {
        // value is equal to node.value
        // At this point, the node to be deleted has been found
        // handle 3 special conditions
  
        // case 1: When the node to be deleted is a leaf node
        if (node.left === null && node.right === null) {
          node = null;
          return node;
        }
  
        // case 2: When the node to delete has only one right child
        if (node.left === null) {
          node = node.right;
          return node;
          // The same deleted node has only one left child node
        } else if (node.right === null) {
          node = node.left;
          return node;
        }
  
        // case 3: When the node to be deleted has two children
        const aux = this.minNode(node.right);
        node.value = aux.value;
        node.right = this.removeNode(node.right, aux.value);
        return node;
      }
    }
  }

  let tree = new BinarySearchTree<number>();
  console.log(tree.getRoot());
  
  tree.insert(11);
  tree.insert(7);
  tree.insert(15);
  tree.insert(5);
  tree.insert(3);
  tree.insert(9);
  tree.insert(8);
  tree.insert(10);
  tree.insert(13);
  tree.insert(12);
  tree.insert(14);
  tree.insert(20);
  tree.insert(18);
  tree.insert(25);
  console.log(tree.getRoot());
  console.log(tree.getRoot().left);
  console.log(tree.getRoot().right);
  
  tree.preOrderTraverse(console.log);
  tree.inOrderTraverse(console.log);
  tree.postOrderTraverse(console.log);
  
  console.log(tree.search(8));
  console.log(tree.search(0));
  console.log(tree.min());
  console.log(tree.max());
  console.log(tree.remove(8));
  console.log(tree.search(8));